Key points of this research results
- The concept of designs on unitary groups is getting attention related to a method of quality tests of quantum gates, which are important component parts of quantum computers.
- However, a method to construct designs with higher strength rigorously had been unknown previously.
- In our research, we give an inductive algorithm to construct designs on unitary designs with higher strength rigorously and explicitly.
Outline
In Combinatorics, which is a researching area of Mathematics, many researchers study ``designs’’. Roughly, a concept of designs is defined as ``balanced’’ point arrangements on some kinds of spaces. More strictly, for each point arrangement, as an indicator of ``balance’’ of the arrangement, a concept of ``strength’’ of it is quantified in terms of integrations. In general, designs with higher strength are useful for some applications. Theoretically, it is known that designs with higher strength exist (proved by contradiction). However, a method to construct designs with higher strength rigorously has been unknown.
Note that concepts of designs depend on the space in which we consider point arrangements. In particular, studies on designs on unitary groups, which are kinds of symmetric spaces, have been deeply developed started with the paper by A. Roy and A. J. Scott in 2009. Furthermore, as a method of quality tests of quantum gates, which are important component parts of quantum computers, the randomized benchmarking using designs on unitary groups are getting attention.
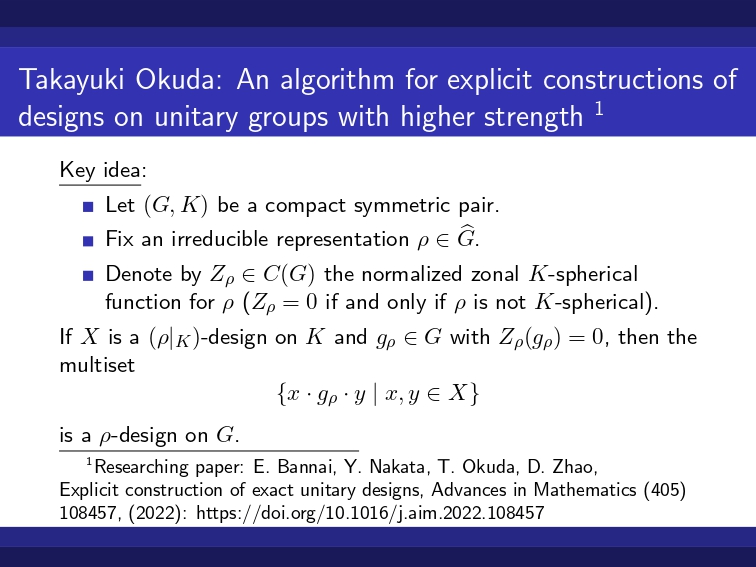
However, even for designs on unitary designs, a method to construct designs with higher strength rigorously had been unknown previously. In our research, by applying representation theory (a researching area of mathematics), we give an inductive algorithm to construct designs on unitary designs with higher strength rigorously and explicitly.
Paper Info
Eiichi Bannai, Yoshifumi Nakata, Takayuki Okuda, Da Zhao, Explicit construction of exact unitary designs, Advances in Mathematics (405) 108457, (2022):
https://doi.org/10.1016/j.aim.2022.108457

 Home
Home



